Twistower part 2 prelimenary test
-
24-Jul-2011
A small scale preliminary test with the Twistower was fulfilled on July 2011. Two types of Twistowers with a 0.3 m diameter were tested, one of 0.9 m and one of 2 m high. Although a very simple quick reference test sample and test rig were used the sample operated well and proves that the concept is robust and promising.
The Twistower is a cost effective floating up draft solar tower made of thin fabric walls.These walls are pre-shaped by inflated helium baleens to make starting possible. Once operational, just as a natural twister, the shape is established by hot air and the centrifugal force on the rotating tower. (Read more about the "Twistower" in the previous "Twistower" article.)
Test rig
For this test the hot air supply was created by a wood fire. To start the Twistower and for it to develop a vortex, a 2 m high static chimney with a vane grid was applied. At the top of the chimney a bicycle wheel is mounted, while the Twistowers are mounted on the wheel as can be seen in figure 1. To avoid burning the test samples are made of thin glass fibre fabric. For an up draft solar tower lighter and stronger fibres will be applied. The applied vane grid, which generated the vortex, is shown in figure 2.

Figure 1: Test rig with 0.9 m high Twistower

Figure 1: Test rig with 0.9 m high Twistower
In principle, to avoid destruction of the vortex by horizontal wind forces, the Twistower should be provided with a pendulum bearing, such that the bearing will bent instead of the tower. However for a first preliminary test it was decided to skip the pendulum bearing by the application of a simple radial bearing and test during calm wind. However the behaviour at stonger wind forces without pendulum bearing will be tested as well.
Test
The wood fire is designed to provide a 70 K temperature rise over the chimney and the Twistowers. This will generate a chimney under pressure of 8 Pa. With a pressure loss of 2,6 Pa the air speed in the chimney will be 1.7 m/s. The tangential speed of the Twistower wall and at the vortex core can be seen in the movies in figure 3 and is 1 m/s, therefor the pitch angle of the total speed at the wall is 60°.
The pitch angle of the total speed can be checked with the signature at the chimney wall in figure 4.

Figure 1: Test rig with 0.9 m high Twistower
With an axial air speed of 1,7 m/s and an effective chimney pressure of 6 Pa the potential power is 0,8 W and with an power coefficient of 0.5 the net power of this small scale Twistower will be 0,4 W. With a tangential speed of 1 m/s (Twistower rotational speed 60 rpm) the centrifugal pressure of the rotating air on the Twistower wall is 0.9 Pa, which should be higher to overcome the chimney's under pressure. Due to this low pressure the fabric is not stretched and flares up. For sufficient stretching the tangential speed should be 1.7 m/s and the pitch angle of the overall speed of the vortex core should be 45°. This is possible with a more effective vane grid, which will be applied in the next test by adjusting the pitch of the vanes.
The 0.9 m high tower could operate at wind speeds up to 5 m/s without severe bending, while the 2 m high tower can only take up to 2 m/s and makes the application of a pendulum bearing really necessary.
Both towers start up automatically because of the rising hot air flowing through the deflated fabric creating the desired tower shape. The static chimney with a height of 2m is sufficient to fill the Twistower with hot air and to start the operation with an inflated tower.
With a sole static chimney the vortex will be destroyed immediately, because it explodes instantly when it leaves the chimney by producing turbulence. This is because a vortex leaving the sole chimney without a rotating tower is bound and will not be in equilibrium with it's surroundings. If a rotating Twistower is present a natural free vortex (as present in an tornado) will be developed. A free vortex is more stable and will be sustained longer. The 0.9 m high test sample had developed a vortex that sustained more than 2 m above the tower., Due to the lack of a pendulum bearing, the 2 m high sample was not stable enough to develop a longer vortex above the tower and was about 2 m long as well. In figure 5 the assumed development of a free vortex created by the Twistower is drawn.

Figure 5: Development of a free sustaining and stable vortex by the Twistower
Due to the development of a free vortex, the actual “tower” diameter will increase, which let the Twistower act as a diffuser, therefor decreasing the axial speed, recovering pressure from the inlet speed of the tower and decreasing the aerodynamic losses beyond. For a diffuser, radial transport of hot air should be possible, which requires an open fabric for the tower wall.
It is assumed that a stable vortex is developed at a height of 5 times the diameter and that the tower will then be virtually >5 times longer.
Preliminary extrapolation of test results
Due to the low diameter and height of the test sample the tower under pressure was low and the aerodynamic losses were high. The aerodynamic losses will decrease with increasing diameter and the tower under pressure will increase with increasing tower height (including the virtual height of the stable vortex beyond the tower).
In figure 6 the Twistower power dependent on the diameter and the height is presented for an temperature difference of 70 K, excluding the virtual elongation of a continuing vortex.
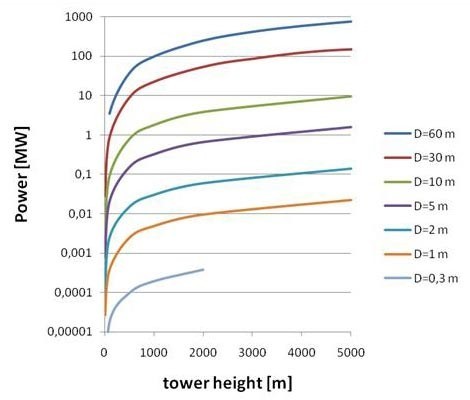
Figure 5: Development of a free sustaining and stable vortex by the Twistower
Due to the lower aerodynamic losses the power increases exponential with the tower diameter and due to the increasing chimney pressure with the tower height .
For better understanding the specific power per tower volume (power divided by π/4 D²H) is shown in figure 7.
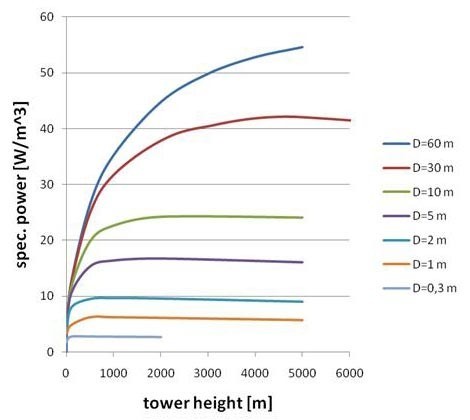
Figure 7: specific power of the Twistower dependent on tower height and diameter
As can be seen, dependent on the diameter above a certain height the specific power is nearly constant and is the power linear with the height. Below that certain height the aerodynamic losses are dominant at lower heights and diameters.
As can be concluded the small scale of the test sample is far from effective and it is a miracle that it did run on an old cycle wheel and a handmade chimney with large gaps at the seals.
Cost consequences
Because the Twistower is floating the tower costs are linear with the wall area. Also important is that the assembly and erection is very cheap. If assumed that the costs are 20 €/m² the costs in €/kW are presented in figure 8.
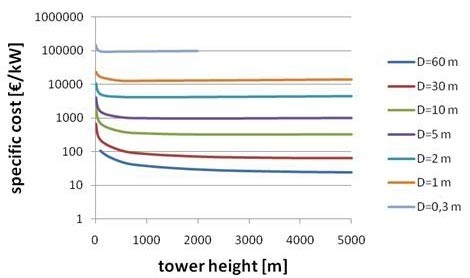
Figure 8: specific cost of the Twistower dependent on height and diameter.
For reasonable costs the Twistower should be larger than 500 m with a diameter larger than 30 m. Smaller diameters are not feasible.
If the vortex continues beyond the tower the costs will go down and even smaller towers will be cost effective. For example the costs of a Twistower with a 20 m diameter is presented in figure 9, dependent on height and continuation of the vortex in tower heights.
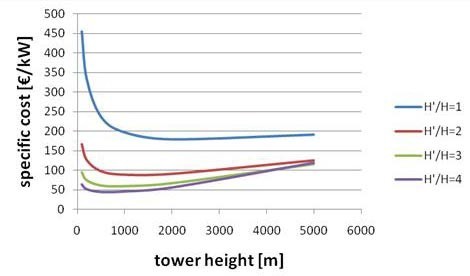
Figure 9: specific costs of a 20 m diameter tower dependent on tower height and virtual elongation by vortex continuation in tower heights (H´/H = virtual height/real height)
At a tower height of 400 m and a virtual height of 2 tower heights the tower costs are already reasonable and will not decrease much if the virtual height is larger. In this case a 20 m diameter 400 m high Twistower is already feasible at a the costs of 100 €/kW.
Twistower with a conventional collector
Because the efficiency of a solar tower is dependent on the tower height, the costs of the collector and turbines should also be implemented to determine the kWh cost. To heat the air of the Twistower a certain collector area is needed. If the costs of this area are estimated at 15 €/m², the costs including the collector can be determined. The costs of the turbines are estimated at 700 €/kW.
In figure 10 the kWh costs of a Twistower with a conventional collector are shown depending on the tower height and the tower diameter.
It is assumed that the mean heating power by the sun is 250 W/m², which is shining 300 days/y (desert) and that the rising air is heated up by 70 K. The yearly costs are assumed to be 14% of the investment cost.
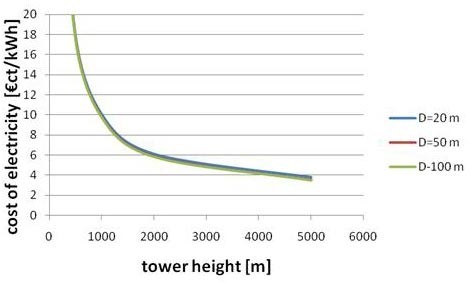
Figure 10: electricity costs of the Twistower with conventional solar collector are dependent on tower diameter and height
Because the collector costs and the turbine costs are dominant the diameter dependency is poor. Reasonable costs of 5 €ct/kWh are reached at a tower height of 3000 m and a diameter of 20 - 100 m.
Because the height dependency is large, the virtual tower elongation by the continuing vortex will be important. To examine this behaviour the elongation in tower heights is presented in figure 11 for a 50 m diameter tower.

Figure 11: electricity costs of the Twistower with conventional solar collector dependent on tower height and virtual tower elongation by vortex continuation (H´/H = virtual height/real height)
The minimum costs go asymptotic to 2,5 €ct/kWh, when the maximum efficiency is reached.
Reasonable electricity costs of 5 €ct/kWh are reached if H´/H = 4 with a tower height of 500 m. If no elongation is present the tower height should be 3000 m.
Twistower with solar pond collector
If a natural solar pond is applied as collector (see also the "Solar pond tower for 5 €ct/kWh" article) with costs of 1 €/m², turbine costs of 700 €/kW and heat exchanger costs of 600 €/kW the costs will be lower than for a conventional collector. The electricity costs of a Twistower with a solar pond as collector are presented in figure 12.
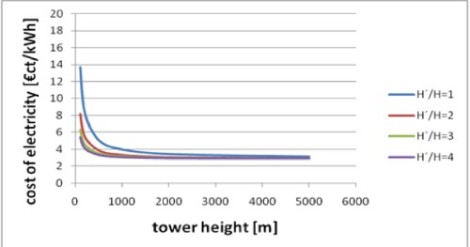
Figure 12: as in figure 11 but now applied for a Twistower with a solar pond as collector
With a solar pond collector very reasonable electricity prices of 3 €ct/kWh are already available for tower heights of 500 m. Reasonable prices of 5 €ct/kWh are available at heigts of 200 m.
Twistower as Hurricane Killer
If used as Hurricane Killer the mean temperature difference is about 10 K, while the hurricane season is about 120 days. However no collector and heat exchangers are required. The electricity price dependent on the tower height for a 50 m diameter tower is shown in figure 13. For the sea cables and anchoring 1000 €/kW will be calculated.

Figure 13: as in figure 11 but now applied for a Twistower as Hurricane killer
If the elongation by the vortex continuation is 4 then a price of 8 €ct/kWh could be reached at tower heights of 300 m. Although the price is higher than for conventional collectors, the Hurricane killer saves a lot of costs because of damage prevention and if subtracted from the investment costs the kWh-price will be lower than for conventional collectors.
Discussion
For towers higher than 500 m and temperature differences larger than 30 K, to compensate for the chimney under pressure, the required tangential speed of the vortex is much larger than the axial vortex speed. Such a vortex cannot be generated by a static vane grid and a cyclone with tangential inlets is needed. Another possibility is the application of intermediate turbines in the tower, which decreases the chimney under pressure below each turbine. Because the axial force on the turbine is larger than the weight of the turbine, the turbine will float and give the tower extra lift. This will give an advantage because less helium will be needed. However the starting and the stopping of the tower will be more complex, because with the tower, the turbines should lift up as well.
In all cases the power consumption for the vortex generation is 16% of the produced power. Due to the enlarged chimney under pressure by the vortex, except for the losses this power will be recovered.
For the Twistower applied as Hurricane Killer (ΔT≈10 K and H<500 m) a static vane grid is sufficient to generate a vortex. For Twistowers applied by low temperature solar ponds this is possible as well. For conventional collectors this is not cost effective and a tangential cyclone or intermediate turbines in the tower should be applied.
Conclusions
Even at a very small scale, where the efficiency is very low the Twistower operates well. At a larger scale the Twistower will then operate excellent.
To improve the stretching of the tower wall the tangential speed of the vortex should be increased by adjusting the pitch of the vanes in the vane grid. Or it is possible to apply a cyclone with tangential inlets, which is also recommended if the tower is > 500 m with temperature differences > 30 K.
A static chimney of 2 m is sufficient to start the Twistower.
Without pendulum bearing the Twistower can run upto wind speeds of 2 m. For higher wind speeds a pendulum bearing is required.
When there is no Twistower mounted on the static chimney, the generated vortex will be destroyed immediate and no twister develops. If a Twistower is mounted, a twister will be present in the rotating tower and it will continue beyond the tower. It can be concluded that the Twistower develops a free vortex that is more stable and will continue beyond.
If a free vortex is developed by the Twistower, it acts as a diffuser by application of a wall with a more open fabric. If applied the aerodynamic losses will decrease and a longer virtual tower with a greater power and efficiency will be generated by the continuation of the vortex.
The costs of the Twistower is orders lower than the costs of conventional towers or chimneys. If applied in solar up draft towers the price of the generated electricity can be as low as 3 €ct/kWh.
Recommendations
Due to the successful test and the towers high potential it is recommended to continue further experiments. In the next phase a larger tower with a pendulum bearing should be made, while for the tower wall, different open fabrics should be tested to examine the diffuser behaviour of the tower and the development and continuation of a stable free vortex.

Figure 14: A dust devil as presented by friendly forcast is generated by hot desert air and is the natural but deterministic elongation of the Twistower
-
24-Jul-2011
